
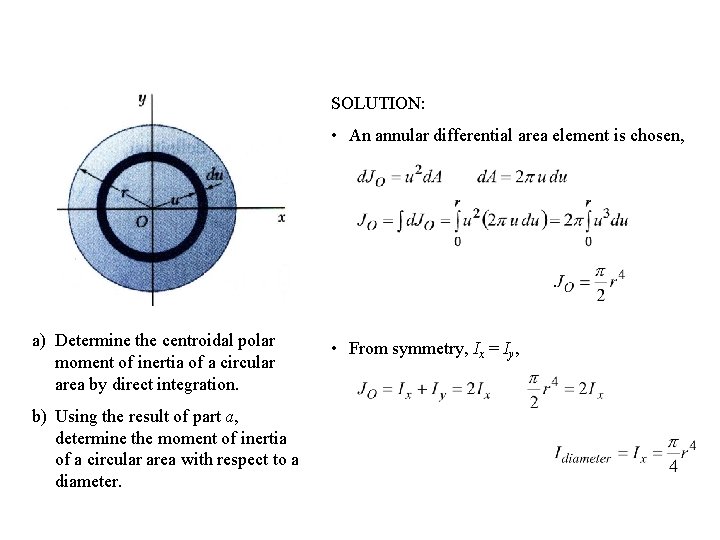
If (x, y) is any point on the circle, then the distance between that point and the centre (a, b) is: This equation can be derived using the distance formula between two points. Where (a, b) are the coordinates of the centre of the circle, and r is the radius of the circle. The equation of a circle in a Cartesian coordinate system can be written as: In analytical geometry, geometric figures such as curves, lines, points, and surfaces are represented using coordinates in a coordinate system. Definition of Radius in Analytical GeometryĪnalytical geometry, also known as coordinate geometry, combines algebra and geometry to study geometric objects using algebraic techniques. The study of the relationships between the angles and sides of triangles, which is the basis of trigonometry, relies heavily on the concept of the radius of a circle. The radius is a fundamental concept in trigonometry, as it is used to define trigonometric functions and to describe the positions of points on the unit circle. The radius of a circle is also used in the polar coordinates system, where the position of a point on the plane is described in terms of its distance from the origin (the radius) and its angle from the positive x-axis. The sine of the angle represents the y-coordinate of the point, the cosine of the angle represents the x-coordinate of the point, and the tangent of the angle represents the slope of the line connecting the origin to the point.
Specifically, if we consider an angle formed by a ray from the origin to a point on the unit circle and the positive x-axis, then the coordinates of the point on the unit circle can be expressed in terms of the trigonometric functions sine, cosine, and tangent. The unit circle is used to define the trigonometric functions of angles. However, in trigonometry, the radius is often used in the context of the unit circle, which is a circle centered at the origin of the coordinate plane with a radius of 1. This definition is similar to the definition of radius in classical geometry. In trigonometry, the radius of a circle is defined as the distance from the centre of the circle to any point on the circle. Trigonometry has many applications in fields such as physics, engineering, architecture, and astronomy, where it is used to model and analyse various phenomena. Trigonometry is a branch of mathematics that studies the relationships between the sides and angles of a triangle. Moreover, the length of the radius is half the length of the diameter, and the circumference of a circle is equal to pi times the diameter or 2 times pi times the radius. For example, all points on a circle are equidistant from the center, which means that the radius is perpendicular to the tangent line at any point on the circle. The radius of a circle has several properties that make it a fundamental concept in geometry. The circumference of a circle, on the other hand, is the distance around the circle. The diameter of a circle, which is twice the length of the radius, is equally important, as it represents the distance across the circle through the center. Thus, the radius is a crucial element of a circle's definition in classical geometry, as it determines the circle's size, shape, and position. The radius is the distance from the center to any point on the circle.

In classical geometry, we define a circle as a set of points in a plane at an equal distance from a fixed point, known as the center of the circle. Definition of Radius in Classical GeometryĬlassical geometry is a branch of mathematics that deals with the properties of geometric shapes in two or three-dimensional space.

We will also discuss the applications of the radius of a circle in various fields and examine its properties and relationships with other geometric concepts. In this article, we will explore the definition of the radius of a circle in different branches of mathematics, including classical geometry, trigonometry, and analytical geometry. Understanding the properties of a circle, and in particular, its radius, is crucial in many areas of mathematics, from geometry to calculus. The circle is one of the most fundamental geometric shapes in mathematics, with a wide range of applications in various fields such as engineering, physics, architecture, and more. Next → ← prev Radius of a Circle Definition


 0 kommentar(er)
0 kommentar(er)
